5 Lab and Medical Math 9/2-9/8
Procedure
A. Molar Concentrations
1) How you would make 500mL of a 0.05M NaCl solution.
2) How much arginine do you need to make a 0.01M solution of arg (MW = 174.20)?
3) How much arginine do you need to make 100 ml of a 0.01M solution of arg (MW = 174.20)?
B. Concentrations weight/volume (w/v)
1) How much NaCl do you need to weigh out to make 1L of a 0.9 % NaCl solution?
C. Diluting a Solution to an Unspecified Volume in Just One Step
1) If you have a 1M arg stock and you want on inject a 100 ml of a 1mM solution– What do you use to dilute the stock?
2) Dilute a solution that has an initial concentration of solute of 10% down to 2%.
3) Dilute a solution that has an initial concentration of 0.01% to 0.0001%.
D. Diluting a Solution to a Specified Volume in Just One Step
1) You have a 0.01% solution. You want a 0.001% solution and you need 25 ml.
2) Prepare 50 ml of a 3% solution from a 4% solution.
3) Prepare 100 ml of 10% alcohol from 95% alcohol.
4) You need 45 ml of 50% alcohol. How can you prepare this from 70% alcohol?
5) What volume of a 20% dextrose broth solution should be used to prepare 250 ml of 1% dextrose broth?
6) Prepare a 45 ml of a 2% suspension of Red Blood Cells (RBCs) from a 5% suspension.
7) What volume of a 0.02% solution can be prepared from 25 ml of a 0.1 % solution?
8) What percent concentration of alcohol is prepared when 10 ml of 95% alcohol are diluted with H20 to make a final volume of 38 ml?
9) What percent of dextrose is prepared when 3 ml of a 10% dextrose solution are mixed with 12 ml of broth?
E. Diluting a Solution to an Unspecified Volume in Several Steps
1) You have a stock solution of protein containing 10 grams per ml. You want a concentration of 2 mg/ml. How would you perform this dilution in just three steps?
2) You have a stock solution of 10 mg/ml of vitamins and want to obtain a solution of 0.5 μg/ml. How would you perform this dilution in just three steps?
F. Diluting a Solution to a Specified Volume in Several Steps
1) You want a 1:128 dilution of serum and you need 4 ml. How would you perform this dilution in several steps?
2) You want a 1:3,000 dilution of serum and you need 2 ml. How would you perform this dilution in several steps?
3) How would you prepare 1 ml of a 1:5 dilution of sera?
4) How would you prepare 8 m1 of a 1:20 dilution of sera?
G. Serial dilutions
1) Based on the following dilutions, how many bacteria were present in the original sample?
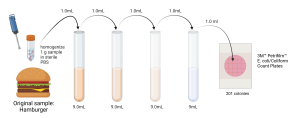
2) How many bacteria were present in the original sample (CFU/ml)?
|
Dilution |
Volume Plated |
Colonies Counted |
|
10-3
|
1.0 ml |
TNTC |
|
10-4
|
1.0 ml |
256 |
|
10-5
|
1.0 ml |
21 |
|
10-6
|
1.0 ml |
7 |
|
10-7
|
1.0 ml |
0 |
|
10-8
|
1.0 ml |
1 |
3) If 0.1 ml of a urine culture from a 10-6 dilution yielded 38 colonies, how many bacteria were there per ml in the original sample?
4) How many bacteria were present in the following sample?
|
Dilution |
Volume Plated
|
Number of Colonies Counted
|
|
10-7
|
0.1 ml |
26 |
5) How many bacteria were present in the following sample?
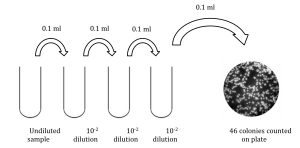
6) How many bacteria were present in the original sample?

Answers and work:





Why is it important?
Accurate calculations in the laboratory and medical fields are essential for ensuring the safety, efficacy, and reliability of experiments, treatments, and diagnoses. Precision in calculations allows for correct dosages, proper experimental conditions, and reliable data interpretation. Inaccurate calculations can lead to harmful outcomes, such as incorrect drug dosages, faulty experimental results, or misdiagnosis, all of which can have serious consequences.
For example, in a case study involving medication administration, an incorrect calculation of drug dosage could lead to a medication overdose or underdose, potentially causing harm to patients. An overdose may lead to toxic effects, while an underdose could result in ineffective treatment, allowing a condition to worsen. In laboratory experiments, improper calculations of reagents, concentrations, or temperatures can skew results, leading to erroneous conclusions that may affect future research or public health recommendations.
In both fields, ensuring accurate calculations is vital for preventing accidents, ensuring patient safety, and maintaining the integrity of scientific and medical work.
